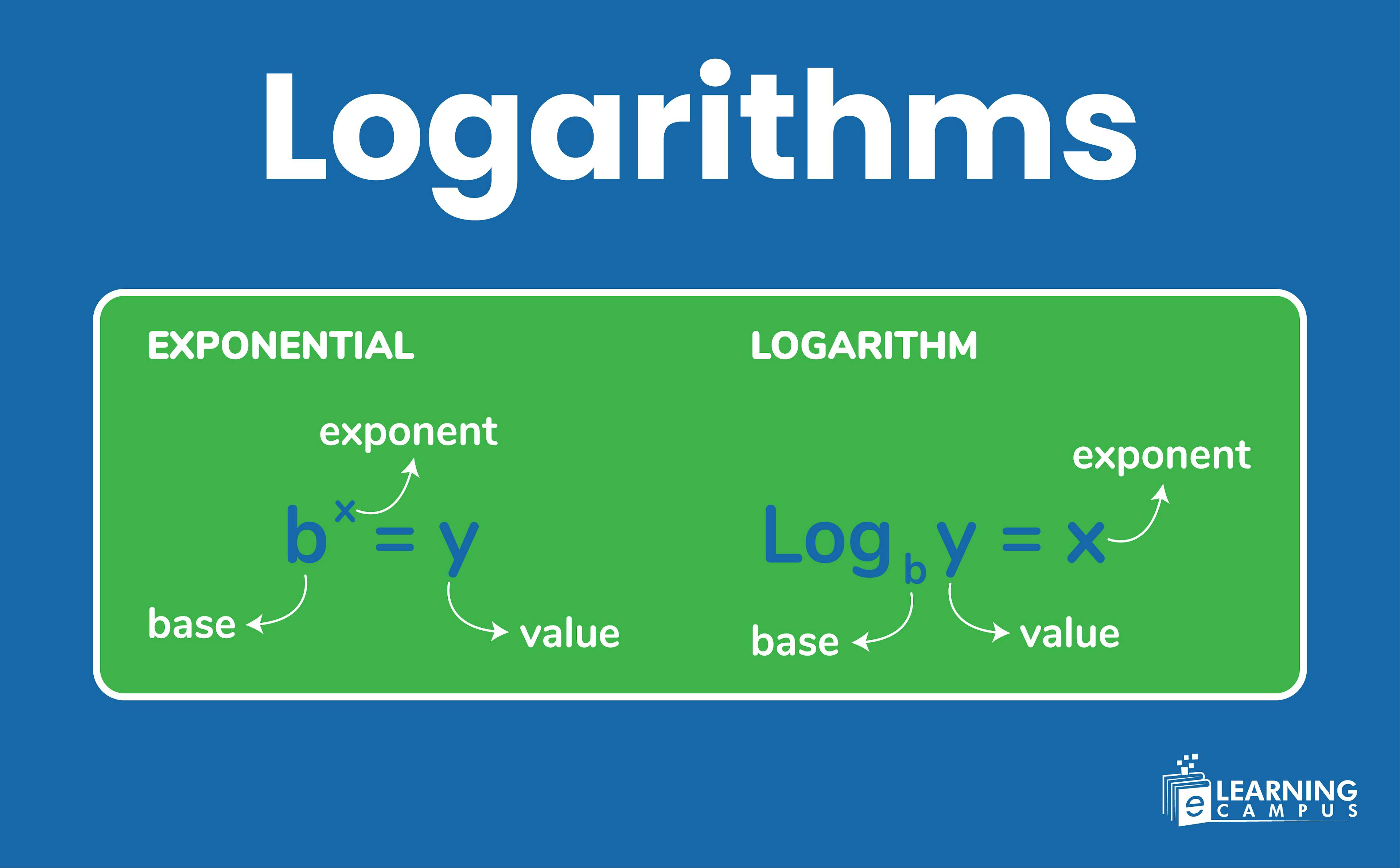
elearning BlogLogarithms- Definition, Properties, and Rules 

Logarithms- Definition, Properties, and Rules 

Logarithms

The logarithm is a quantity that represents the power to which a fixed number is raised to produce a given number. In Mathematics, logarithms are a unique way to express an exponential form of equation in a simple form. It can also be understood as the inverse operation of exponentiation. 

In this blog, you will learn about logarithms in detail with the formula, rules, and properties. 

What are Logarithms

Mathematics has unique inverse rules. Just as division is the inverse of multiplication, logarithms are the inverse of exponentiation. In simple terms, a logarithm answers the question, ‘To what power must a base number be raised to get a specific number?’

For example, if 2 raised to the power of 3, it equals 8, i.e, 2³ = 8, then the logarithm of 8 is 3, it will be expressed as;

log₂8 = 3   

Standard Form of Logarithm 

The logarithmic form is a rearrangement of an equation of exponential form. In this form, the same numbers are written in a different order. For the exponential form ab=c, the standard logarithmic form is expressed as;

log⁡a(c)=b\log_a(c) = b
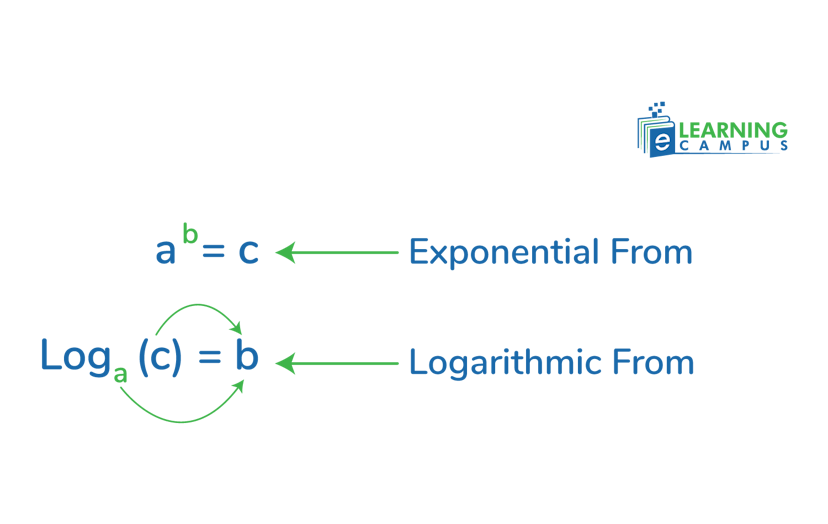
Standard form of logarithms

To understand the concept of logarithms, we will learn the basic terms associated with this concept. 

  • Log: The log in the equation represents a logarithm. 
  • Base: The base is a number that is written in subscript next to a log. 
  • Exponent: The number that is written after the equation symbol is the exponent. 

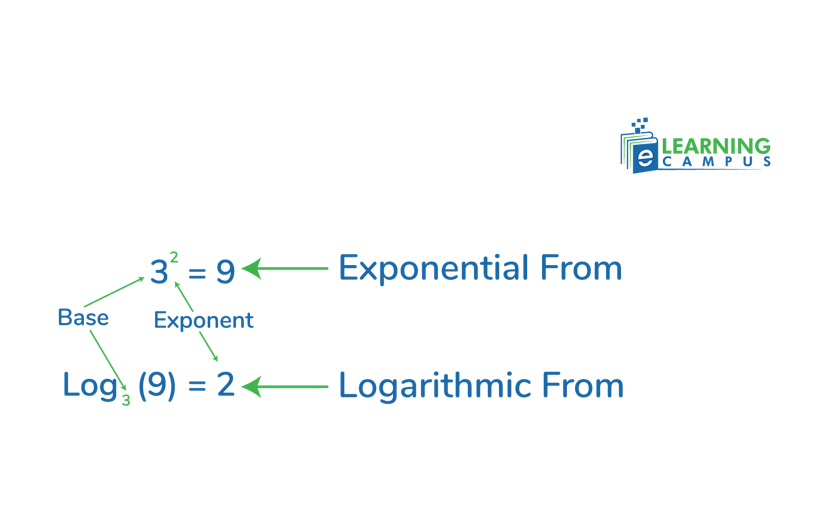
Let’s look at the example of how exponential form is written in logarithmic form.

We know that

39=23^{9} =2

The equation in the log form will be written as;

log⁡3(9)=2\log_3(9) = 2
Logarithm example

Types of Logarithms 

There are three main types of logarithms in Mathematics, namely the common logarithm, the natural logarithm, and the binary logarithm. Let’s discuss each in detail. 

Common Logarithm 

A common logarithm is also known as a decadic logarithm, decimal logarithm, or Briggsian logarithm. It is written with base 10. 

It is often written as log(x), where x is the number whose logarithm is to be taken. A log without a base means it is log 10. 

log⁡10=log⁡\log_{10} = \log

For example, 

log⁡(1000)=3\log(1000) = 3

It means that if we multiply the number 10 three times, we get 1000. In logarhythmic form, it is expressed as;

103=1000⇒log⁡101000=3(or simply)log⁡1000=310^3 = 1000 \quad \Rightarrow \quad \log_{10} 1000 = 3 \quad \text{(or simply)} \quad \log 1000 = 3

Natural Logarithm 

The natural Logarithm is known as the base e logarithm. It is represented in the form;

lnorlog⁡eln or \log_e
log⁡e=ln⁡\log_e = \ln

In this log, ‘e’ represents Euler’s constant, whose value is equal to 2.71828. 

Let’s look at the examples

ex=3⇒ln⁡3=xe^x = 3 \quad \Rightarrow \quad \ln 3 = x
ln⁡3=x\ln 3 = x

Binary Logarithm 

A binary logarithm is the logarithm to the base 2 or log 2. It essentially inquires that "To what power must 2 be raised to obtain a certain number?" It is represented as;

log⁡2x\log_2 x

or

$$ \log_b x $$

Rules and Properties of Logs

The logs possess certain properties that are based on the exponent rules. These properties are also known as the rule of logs. Let’s discuss each rule in detail. 

Product rule 

The product rule of logs states that ‘the product of two logarithm values is equal to the sum of the individual logs. It is expressed as;

log⁡b(mn)=log⁡bm+log⁡bn\log_b (mn) = \log_b m + \log_b n

Example,

log⁡2(3y)=log⁡2(3)+log⁡2(y)\log_2 (3y) = \log_2 (3) + \log_2 (y)

Division Rule

The logs’ division rule states that ‘the division value of two logs equals the difference between the two logarithmic values. 

log⁡b(mn)=log⁡bm−log⁡bn\log_b \left( \frac{m}{n} \right) = \log_b m - \log_b n

For example,

log⁡2(3x)=log⁡23−log⁡2x\log_2 \left( \frac{3}{x} \right) = \log_2 3 - \log_2 x

Exponential Rule

The exponential rule states that ‘ the log of m with an exponent that is a rational number is always equal to the exponent times of its logarithum. 

log⁡b(mn)=nlog⁡bm\log_b (m^n) = n \log_b m

Example,

log⁡b(32)=2log⁡b3\log_b(3^2) = 2 \log_b 3

Change of Base Rule

The change of base rule for logarithms states that the logarithm of a number m to the base b can be expressed as the logarithm of m to a new base divided by the logarithm of b to the same new base a. It gives rise to logarithms of different bases. 

log⁡bm=log⁡amlog⁡ab\log_b m = \frac{\log_a m}{\log_a b}

Example, 

log⁡b3=log⁡a3log⁡ab\log_b 3 = \frac{\log_a 3}{\log_a b}

Negative Log Property

A log with a value less than 1 is a negative log. To convert a negative log into a positive one, we need to take the reciprocal of it.

−log⁡ba=log⁡ba−1=log⁡b(1a)-\log_b a = \log_b a^{-1} = \log_b \left( \frac{1}{a} \right)
−log⁡ba=log⁡b(1a)-\log_b a = \log_b \left( \frac{1}{a} \right)

The Log of 1

The value of log 1 is always zero, irrespective of base. We know that a0 = 1.

If we convert it into a log form, it will be expressed as;

log⁡a1=0\log_a 1 = 0

Solving Word Problems Using Logarithms

As we have learned the logarithm in detail. Now we will solve the word problems using logarithms. Let’s look at some of the examples.  

Example 1

If log 3 = 0.2010 and log 4 = 0.3771, then using the properties of log, find the value of log 36.

log36=log(32×42)log 36 = log (3^2 × 4^2)

By using the product rule,

log32+log42log 3^2 + log 4^2
2log3+2log42 log 3 + 2 log 4

Putting the values of log 3 and log 4

2(0.2010)+2(0.3771)2(0.2010) + 2(0.3771)
0.4020+0.7540.4020 + 0.754
log36=1.156log 36 = 1.156

Example 2

Find the value of  x in

log⁡2x=5\log_2 x = 5

In exponential form, it can be written as;

25=x2^5 = x
x=2×2×2×2×2=25=32x = 2 \times 2 \times 2 \times 2 \times 2 = 2^5 = 32
x=32x=32

Conclusion 

Logarithms are an important concept in Mathematics. They are a unique way to write exponential form in its inverse. It helps to solve complex equations with exponents. 

Make Math Fun With Us

Are you struggling with mathematical concepts and want to learn them in a fun way? We are at your service. We have expert online math tutors who make math easy for you and your kids. You will be the math champion in less time.

Live math tutor
Hire a tutor now 

FAQs